|
|
2012/08/06 2012/08/08 2012/08/11 2012/08/13 2012/08/15 2012/08/17 2012/08/19 2012/08/20 2012/09/10
|
|
MPC observation computed with Astrometrica for plate constants and improved in IDL using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work, the following given magnitude calculated with a circular aperture radius = 5 arcsec:
As the next step, magnitudes enclosed by aperture radii varing from 1-pixel to 10-pixel in a step of 0.5-pixel were calculated in IDL by aper.pro with the solved optocenter pixel coordinates of the comet found by routine cntrd.pro. The inner sky annulus was set to 20-pixel, while the outer one was 30-pixel. I had intended to obtain magnitudes enclosed by larger apertures, however, a bright star was situated too close to the comet and it would not be an easy task to remove the contamination completely, whereby error would definitely be increased largely. Therefore I would rather rely on predicted value yielded by some reasonable empirical equation. The results are listed as follows:
I attempted to fit the magnitude data by the following model funciton,
$ m\left ( r \right )=m_\infty -2.5\log \left [ 1-\exp \left ( -\frac{r}{\rho} \right ) \right ] $ (1),
where $ r $ is the radius of some aperture, $\rho $ is the apparent radius of the coma, and $ m_\infty $ is the magnitude obtained by an infinite aperture. However, I found that the measured diameter was far from sufficience if one hopes the model validates. Only by assuming a diameter in an order of several degrees could make the fitting reasonably close to the data, which was quite impractical for such a dimming comet. I thereby arbitarily presumed a new parameter $n$ tweaking Equation (1):
$ m\left ( r \right )=m_\infty-2.5\log \left [ 1-\exp \left ( -\left( \frac{r}{\rho} \right) ^n \right ) \right ] $ (2),
After several tests, $n = 1.465$ was found to best describe magnitudes in different apertures, probably indicating an alternative distribution of intensity of the coma vs radius to the central coma, i.e., pseudonucleus. Now I can obtain $m_\infty = 10.496 \pm 0.073$, consistent with outcomes from visual observers.
The parameter $n$ is a dependent upon the coma diameter in the way I defined. So long as the diameter is found larger, $n$ will necessarily be smaller to fit the data. There is a strong likelihood that $n$ is actually smaller than what I found here since the exposure was too short to reveal a complete coma of the comet. To make matters worse, the comet was about to set behind an obstruction building so a large portion of the signal from the comet has been blocked. The vignetting function of the image was not correctly performed as well, which worsens residuals.
Copyright @ Man-To Hui 2012/08/08
|
|
I attempted to capture this comet by myself through seriously light-polluted western sky along with some haze. Only by stacking a number of images could I spot some hints of the comet. Even though, the SNR was far from sufficience, and consequently errors in photometry and astrometry were inevitably large.
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
As the next step, magnitudes enclosed by aperture radii varing from 2-pixel to 16-pixel in a step of every single pixel were calculated in IDL by aper.pro with the solved optocenter pixel coordinates of the comet found by routine cntrd.pro. The inner sky annulus was set to 20-pixel, while the outer one was 30-pixel. However, I forgot to save the results.
The magnitude fitting was accomplished as follows:
The parameter $n$ eluded me since I failed to save the consequence. I can recollect that it was not too far from the $n$ I obtained during previous observation from C42, quite consistent instead.
Copyright @ Man-To Hui 2012/08/21
|
|
| Images stacked in median. Mouse-over to browse the image in 4-color sequels version. |
|
|
| Images stacked in average. Mouse-over to browse the image in inverted 4-color sequels version. |
MPC observation computed with Astrometrica:
As the next step, magnitudes enclosed by aperture radii varing from 1-pixel to 24-pixel in a step of every single pixel were calculated in IDL by aper.pro with the solved optocenter pixel coordinates of the comet found by routine cntrd.pro. The inner sky annulus was set to 30-pixel, while the outer one was 40-pixel. However, unfortunately I forgot to preserve the photometric measurements and therefore I could not present lists of value here.
I attempted to fit the magnitude data by Equation (2):
Yet again I failed to save the value of $n$ and now I have already forgotten how large it actually was for best fitting. Nonetheless, I remember clearly that it satisfies $ 1 < n < 2$. It seemed that the integrated magnitude was much smaller than previous observations, thus being much brighter, which might hint a potential outburst to the comet. However, as the geometric observing condition was only poor, one cannot draw any firm conclusion unless more observations come.
Copyright @ Man-To Hui 2012/08/21
|
|
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
As the next step, magnitudes enclosed by aperture radii varing from 1-pixel to 24-pixel in a step of every single pixel were calculated in IDL by aper.pro with the solved optocenter pixel coordinates of the comet found by routine cntrd.pro. The inner sky annulus was set to 30-pixel, while the outer one was 40-pixel. The results are listed as follows:
I attempted to fit the magnitude data. $n = 1.27$ was found to best describe magnitudes in different apertures, yielding $m_\infty = 10.322 \pm 0.075$.
Copyright @ Man-To Hui 2012/08/21
|
|
I attempted to capture this comet by myself through seriously light-polluted western sky. Only in stacked images did the comet show up faintly. Errors in photometry and astrometry were inevitably very large.
MPC observation computed with Astrometrica using UCAC-2 catalog:
Attributed to the extremely low SNR, I did attempt to apply to measure photometry of the comet in a final stacked image, however, the error turned worse dramatically as apertures increased. Additionally, even the stacked image did not present the morphology of the comet and therefore it would be meaningless to find out suitable parameters to extrapolate how bright the comet was in infinity aperture.
Copyright @ Man-To Hui 2012/08/23
|
|
| Observation in the course of NSP Program. Images stacked in average. Mouse-over to browse the image in inverted 4-color sequels version. |
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
As the next step, magnitudes enclosed by aperture radii varing from 1-pixel to 10-pixel in a step of 0.5-pixel were calculated in IDL by aper.pro with the solved optocenter pixel coordinates of the comet found by routine cntrd.pro. The inner sky annulus was set to 20-pixel, while the outer one was 30-pixel. Larger apertures enclosing the optocenter of the comet were contaminated with fluxes from stars. The results are listed as follows:
I attempted to fit the magnitude data then. $n = 1.50$ was found to best describe magnitudes in different apertures, whereby $m_\infty = 10.551 \pm 0.053$ was yielded.
Shortly after the NSP observation, SASP performed another observation of the comet.
|
|
| Mouse-over to browse the image in 4-color sequels version and L-S filter attached. |
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
Photometry result computed with FOCAS II, which processes images based upon the .LOG file of Astrometrica, by means of Multibox method:
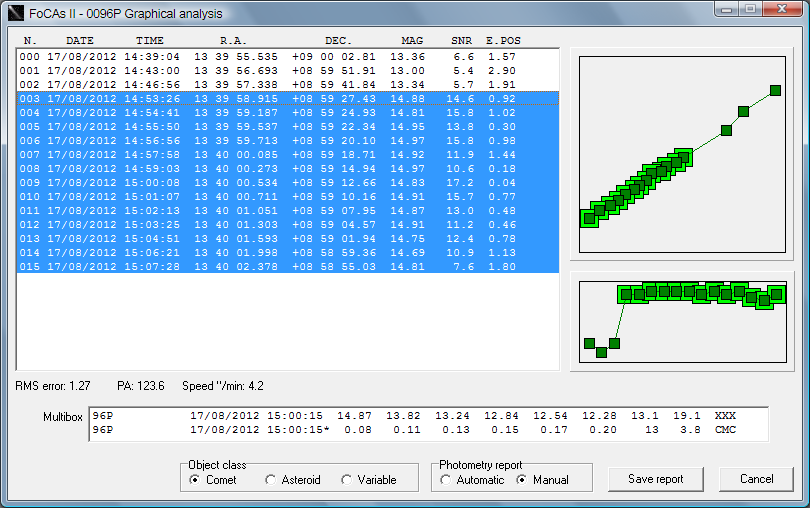
Prior to I exploiting IDL for infinity magnitude fitting, I measured the diameter of the coma, the value of which could be seen in the above image. I did not convert the flux that would have been in corresponding circular apertures from the flux enclosed by square boxes in the same dimension. With several tests, I obtained that $n = 1.37$ best describes magnitudes in different square boxes, thereby $m_\infty = 10.36 \pm 0.01$.
Despite that circular apertures were done for NSP observations whilst square boxes were for SASP observations, adding that different setup was employed, $m_\infty$ is almost consistent with each other.
Copyright @ Man-To Hui 2012/08/21
|
|
| Unfortunately the comet was imaged near the edge of the sequence. Weather was unsteady as well. Stacked in average. Mouse-over to browse the image in 4-color sequels version and L-S filter attached. |
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
Photometry result computed with FOCAS II, which processes images based upon the .LOG file of Astrometrica, by means of Multibox method:
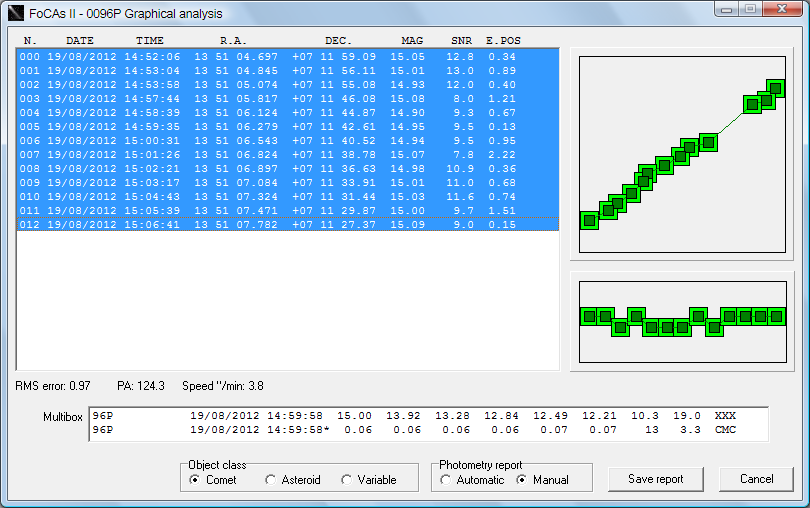
Prior to I exploiting IDL for infinity magnitude fitting, I measured the diameter of the coma, the value of which could be seen in the above image. I did not convert the flux that would have been in corresponding circular apertures from the flux enclosed by square boxes in the same dimension. With several tests, I obtained that $n = 1.32$ best describes magnitudes in different square boxes, thereby $m_\infty = 10.08 \pm 0.01$.
Copyright @ Man-To Hui 2012/08/21
|
|
| Mouse-over to browse the image in 4-color sequels version and L-S filter attached. |
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
Photometry result computed with FOCAS II, which processes images based upon the .LOG file of Astrometrica, by means of Multibox method:
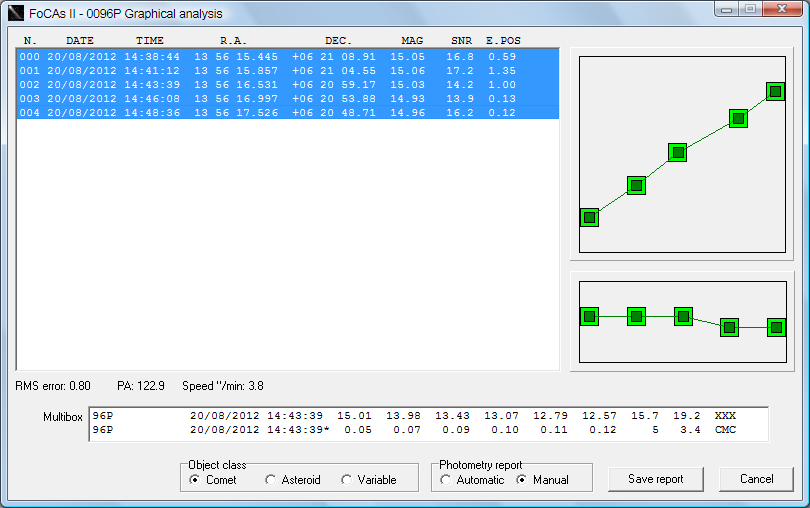
Prior to I exploiting IDL for infinity magnitude fitting, I measured the diameter of the coma, the value of which could be seen in the above image. I did not convert the flux that would have been in corresponding circular apertures from the flux enclosed by square boxes in the same dimension. With several tests, I obtained that $n = 1.30$ best describes magnitudes in different square boxes, thereby $m_\infty = 10.66 \pm 0.03$.
Copyright @ Man-To Hui 2012/08/21
|
|
| Mouse-over to browse the image in 4-color sequels version. |
MPC observation computed with Astrometrica using both UCAC-3 and CMC-14 star catalogs -- the former for astrometric reduction and the latter for photometric work:
- COD C42
OBS M.-T. Hui, X. Gao
MEA M.-T. Hui
TEL 0.36-m f/6.9 Schmidt-Cassegrain + CCD
NET UCAC-3
0096P KC2012 09 10.58628 15 14 07.31 -06 18 23.0 16.5 N C42
0096P KC2012 09 10.59039 15 14 08.02 -06 18 30.1 16.5 N C42
0096P KC2012 09 10.59458 15 14 08.84 -06 18 37.2 C42
----- end -----
Photometry result computed with FOCAS II, which processes images based upon the .LOG file of Astrometrica, by means of Multibox method:
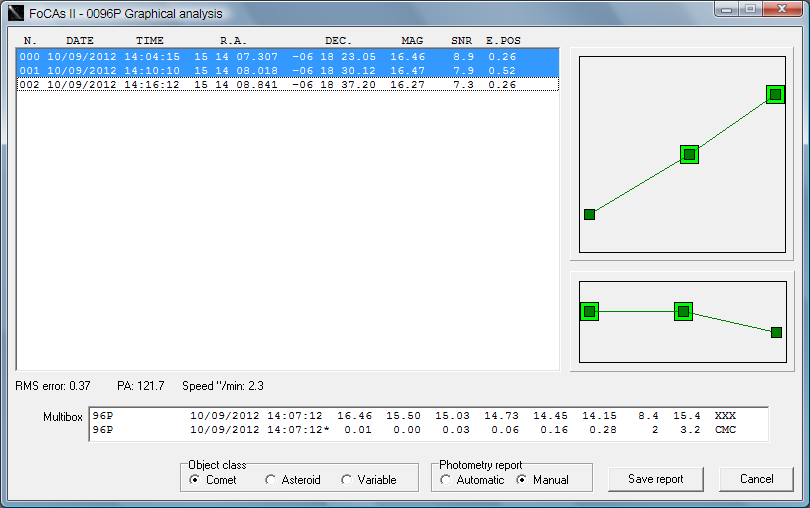
Prior to I exploiting IDL for infinity magnitude fitting, I measured the diameter of the coma, the value of which could be seen in the above image. I did not convert the flux that would have been in corresponding circular apertures from the flux enclosed by square boxes in the same dimension. With several tests, I realized that the coma diameter was too small, possibly due to the diffuse outlook of the comet. I applied the value of 1.0-arcmin from the latest visual observation of the comet, and obtained that $n = 1.47$ best describes magnitudes in different square boxes, thereby $m_\infty = 13.53 \pm 0.06$.
Copyright @ Man-To Hui 2012/09/12
Feel free to e-mail me with any doubts or questions.